The Lab History
In 2004 two research groups working in the field of Quantum Magnetometry were merged together: Prof. R.A.Zhitnikov group developing He-Me magnetometers in A.F.Ioffe PTI since early 70-es, and Prof. E.B.Alexandrov lab developing alkaline magnetometers in S.I.Vavilov State Optical Institute, and since 1999 - also in A.F.Ioffe PTI. This last group experience goes back to 60-es when the project of a zero-field magnetometer, or Hanle magnetometer, was suggested by the today's team scientific leader, member of the Russian Academy of Science Prof. E.B.Alexandrov. The united group works now in A.F.Ioffe PTI in tight cooperaion with S.I.Vavilov SOI. By now it has designed at least six new types of quantum magnetometer of unique features; some of them are listed below.

FIG.1. I.V. Fadeev, I.B. Komissarov, E.B. Alexandrov (SOI) with Potassium Magnetometers on the drifting ice-floe in the North Pole vicinity (1989)



Quantum Magnetometery Overview
Quantum magnetometry is a scientific discipline investigating Earth magnetic field, its time and spatial variations, and magnetic field of various objects on the Earth field background. Sensitivity, accuracy and resolution achieved in quantum magnetometry is extremely high – as one can see, typical relative resolution of magnetometric methods is 10-10 - 10-11. It can only be compared to the time and frequency standards resolution: the quantum magnetometers and the frequency standards use the same basic principles, with the only difference that the quantum magnetometers use magnetic-dependent transitions in atomic structure.


Roughly speaking, all the magnetometric devices may be grouped to two main classes, which are classical and quantum devices. The classical devices are mechanical devices, like magnets suspended on the thin thread, induction coils, etc. There are two types of quantum devices: super-conductive magnetometers based on Josephson effect, and magnetometers using the effect of atomic or nuclear magnetic resonance. The wide-spread proton magnetometers and their Overhauser versions are the simplest devices of this kind. The most advanced devices of the kind are optically pumped quantum magnetometers, realized on different atoms and various atomic transitions; the most popular and wide-spread devices are based on Cesium, Rubidium and Helium, and the most prospective of these device is the magnetometer on super-narrow line of Potassium, or Potassium Magnetometer (See Fig.2).

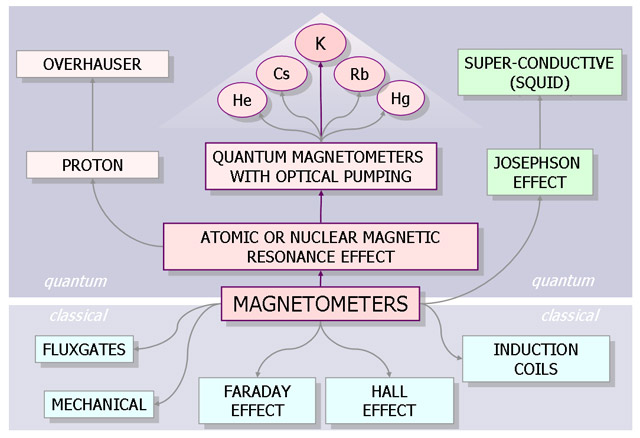FIG.2. CLASSIFICATION OF MAGNETOMETRIC DEVICES BY THE BASIC PRINCIPLES

Comparing these devices is rather complicated task: first, there are many metrological and technical parameters which have to be compared, and second, there is no reliable data in scientific publications since there is no commonly adopted standard procedure of measuring these parameters. More than that, different magnetometric devices measure different physical values – the fluxgates measure the components of magnetic induction vector, the quantum atomic magnetometers measure magnetic induction scalar value, and SQUIDs (as well as induction coils) measure the increment of magnetic flow through some surface, which has to be re-calculated to the magnetic induction increment.


Nevertheless, we have made an attempt to build a very approximate graph (Fig.3) giving some impression about magnetometers distribution by the main metrological parameters – accuracy, sensitivity, and time response. The diagonal arrow shows the general direction of evolution – from the bad to the good (leaving the ugly aside). According to this graph, the Potassium Magnetometer keeps the pole position.

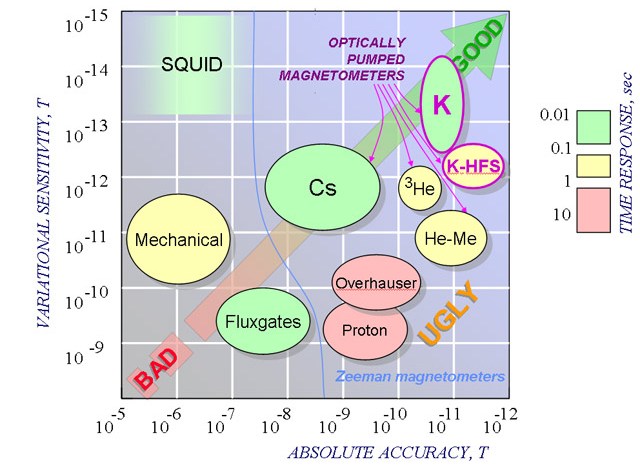FIG.3. QUANTUM MAGNETOMETERS: DISTRIBUTION BY THE MAIN METROLOGICAL PARAMETERS


So why one should need to develop all these devices instead of choosing one the best? The answer lies here, in this diagram showing the Quantum Magnetometry applications (Fig.4). As one can see, there are lots of this applications and they are quite different. The most important of them are physics, geophysics, and geology, but the applications of the Quantum Magnetometry in biology, medicine, and military are swiftly growing.

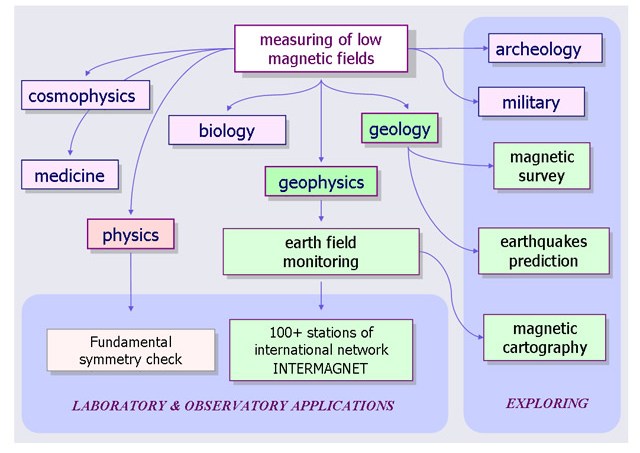FIG.4. APPLICATIONS OF QUANTUM MAGNETOMETRY




Quantum Magnetometers Basic Principles
All the magnetometers designed in the laboratory use optical pumping and double radiooptical resonance techniques, which were briefly described in Optical Orientation Section.

There are three main processes which take place in atomic magnetometer (Fig.5):
  1. Optical pumping, - the process creating the population difference between Zeeman sublevels. The most frequently used kind of the optical pumping is the polarization pumping shown on the diagram. The result of interaction with circularly polarized light resonant to the optical atomic transition is emptying of all Zeeman sublevels save for one.
  2. Magnetic resonance. In terms of atomic populations it means that the radio-field resonant to the atomic transition would equalize the levels population, destroying the population difference. It is important that the resonant field affects not only the levels population, but also their coherence.
  3. Optical detection. The effect of the magnetic resonance can be detected with resonant light – for example, the same light which we use for optical pumping. The main advantage of this method is in its sensitivity – for each radio-frequency photon absorbed by the atom we can detect the absorption of one optical photon which has many orders of magnitude higher energy.

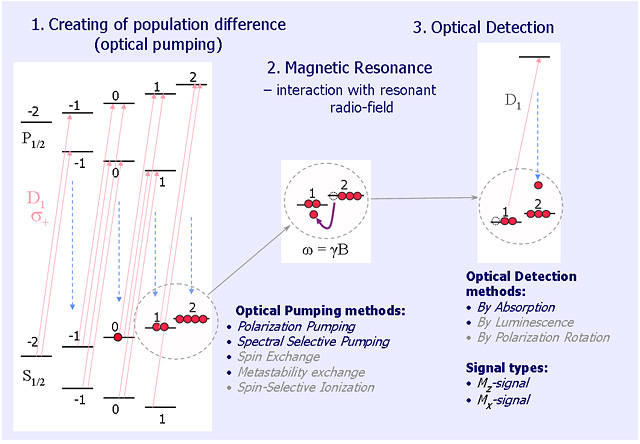FIG.5. THREE BASIC PRINCIPLES OF OPQM
(OPTICALLY PUMPED QUANTUM MAGNETOMETER)


The ground-state of an alkali atom is a complex structure, and the transitions of many kinds can be observed in it; all this transitions (single-photon, multi-photon, Zeeman, hyperfine) we have used in the new magnetometric schemes.

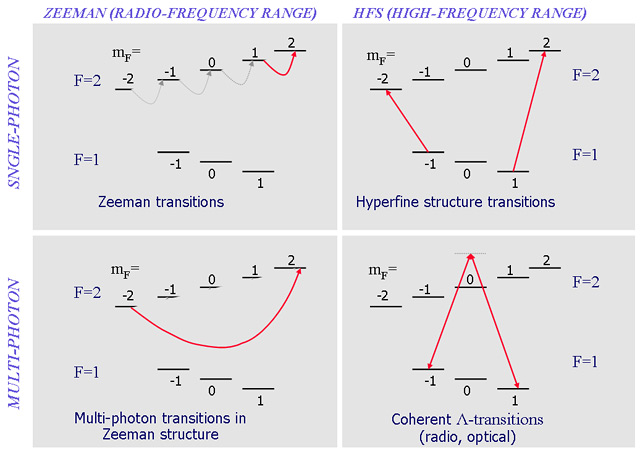FIG.6. TRANSITIONS IN ALKALINE GROUND-STATE
USED IN MAGNETOMETRIC SCHEMES
The magnetometer being developed in the lab most intensively at the moment is Potassium (K) Optically Pumped Mx-Magnetometer. Fig.6 shows potassium spectral structure.



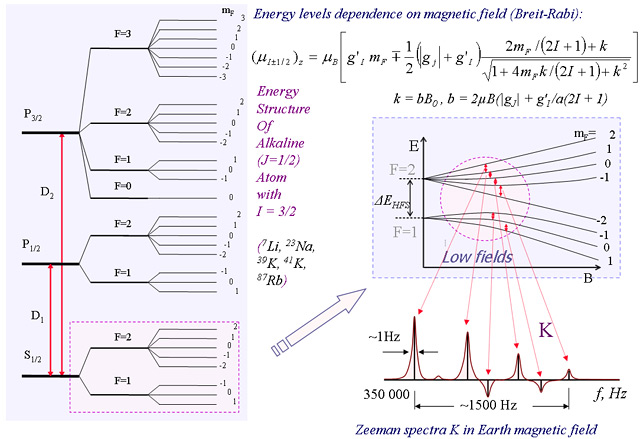FIG.7. BASIC PRINCIPLES OF POTASSIUM MAGNETOMETER


Fig.8 shows the comparison of Cesium and Potassium as working substances for Mx-magnetometer:
Cs: Structure is non-resolved in Earth magnetic field;
Resonances are converged in wide asymmetric line (~15 nT width);
The position of the line maximum depends on the device orientation, optical pumping condition, and so on, which leads to the errors of Cesium magnetometers.

K: Structure is fully resolved in Earth magnetic field;
Spectrum consists of narrow symmetric lines (~ 0.2 ÎôÌ wide), so the line tops positions are quite stable.
K was not used in magnetometry for a long time because of the complexity of its spectrum. The first attempt was made in France in 60-es, but the first device was made in our lab in mid-80es. Still, Cesium device is the most widespread optically pumped magnetometer



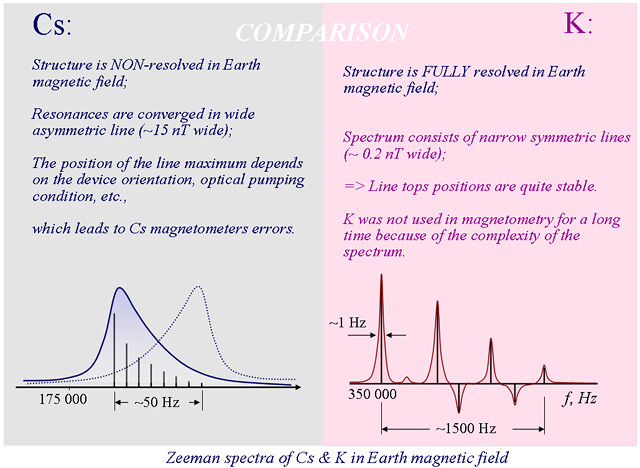FIG.8. COMPARISON OF MAGNETIC RESONANCES IN CS AND K


On Fig.9 the simplest optically pumped magnetometer is shown – it consists of the spectral lamp, which gives light for optical pumping, the cell containing alkaline vapors, the photo-detector, and the electronics which amplifies the Mx resonance signal, turns its phase and applies it again to the cell, closing in this way the feedback loop. The system generates signal at atomic resonance frequency – therefore it is called «Atomic Spin Generator». The system like this can not work with Potassium atoms without special precautions since it would oscillate on several potassium resonance frequencies. Actually, the only way to measure the potassium frequency reliably is to analyze potassium signal using fast microprocessor and processor-controlled low noise synthesizer.



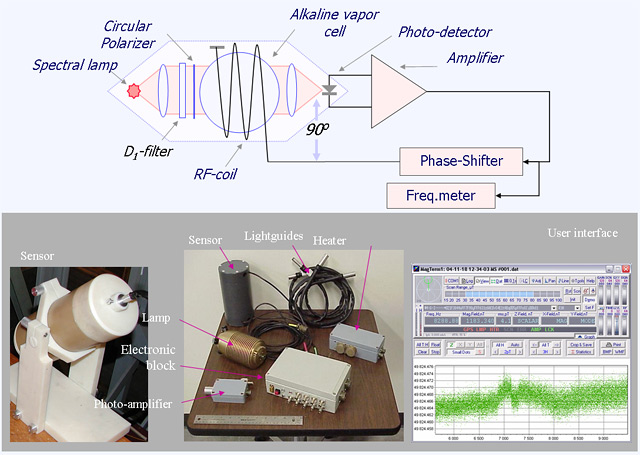FIG.9. QUANTUM MAGNETOMETER WITH OPTICAL PUMPING
(THE SIMPLEST SELF-OSCILLATION VERSION)


Our Non-Self-Oscillating Optically Pumped Potassium Mx-Mag Parameters are:
  • Ultimate sensitivity ~2·10-15 T·Hz-1/2
    (in the sensor with laser pumped O15 cm cell)
  • Typical sensitivity ~5·10-13 T·Hz-1/2
    (in the sensor with lamp pumped O5 cm cell)
  • Absolute accuracy: ~2·10-11 T
  • Time response: ~1·10-3 s


VECTOR MAGNETOMETRY is nother field of our researches. Vector quantum optically pumped magnetometers basically are scalar sensors placed in a coil systems which produce a variable magnetic field.

These device is not the first one based on this principle - instruments like this were created before on the base of the proton magnetometer. But in that case the field in the coil system must be switched several times in order to complete one measurement. The main drawback of this device is that it is slow and therefore it needs very quiet field conditions.

Basically, our concept is:
- We use Quantum Mx-Magnetometer as a scalar sensor, since it is much more sensitive and may be thousand times faster than Proton one (its typical bandwidth is 5 kHz or more).
- Instead of switching an extra field we rotate it with relatively high frequency (up to several hundred Hz).
- We use weak DC extra fields to compensate for the Earth field variations.

The variometer consists of a scalar sensor placed into the center of 3D coil system aligned along EMF. The coil system produces the transverse field rotating at several hundred Hz in the plane perpendicular to the main device axis. The scalar sensor measures the total magnetic field H in the center of the coil system. If EMF vector is parallel to the coil system axis, the length of this vector remains constant during the rotation period.

Let now the EMF vector deviate from the Z-Axis in Y direction: as you can see the oscillating components appear in total field module. It also happens if the EMF vector deviates from the Z-axis in X direction, but in this case the phase of oscillating component appearing in H is shifted by Pi/2 comparing to the previous case. It means that we can detect these oscillating components separately and use them as signals for the X-Y feedback systems. The feedback systems produce transverse DC magnetic fields which would compensate for the field variations until vector signal disappears. Knowing these DC field, we know the EMF transverse variations.



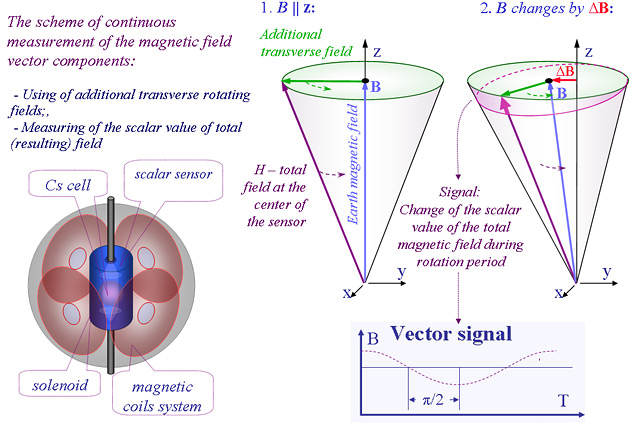FIG.10. FAST 3-COMPONENT VARIOMETER BASED ON A CESIUM SENSOR


Fig.11 shows the results of the tests taken in magnetic field stabilizer, and (with new compact version of the variometer) in the magnetic shield. Taking into consideration stabilizer noise, we can estimate the variometer noise: it constitutes 10-12 pT for a measurement time of 0.1 s.



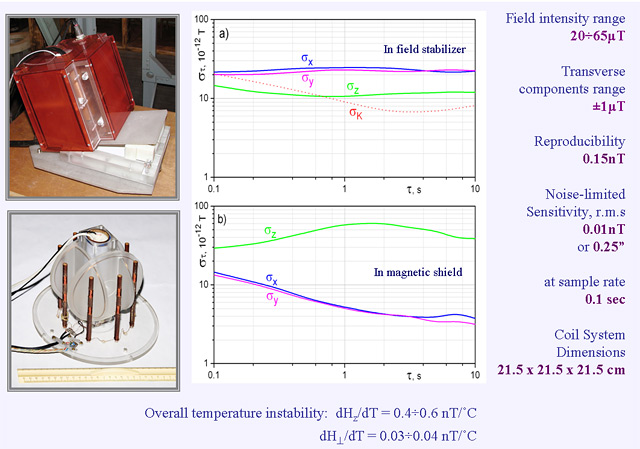FIG.11. FAST 3-COMPONENT VARIOMETER BASED ON A CESIUM SENSOR


Quantum Magnetometers Designed in the Lab

Alkali-helium magnetometer, invented by Prof. R.A.Zhitnikov group, can be implemented only as Mz-scheme. It uses the effect of spin polarization transition during atomic particle collisions in alkali-helium gas discharge plasma. The working substance of this magnetometer is helium in metastable state. An important achievement is the possibility of orienting spin momenta of helium metastable atoms in the absence of direct optical pumping with resonance helium light, which permits the elimination of light shifts in the frequency of magnetic resonance. This magnetometer was quite popular in 1980s as a reference instrument; but today it is inferior to the potassium narrow-line magnetometer in respect of sensitivity, time response, and accuracy.


Self-generating helium magnetometer for measurement of a geomagnetic field with sensitivity 0.01 Îô in a strip of registration of 0.02-0.7 Hz. For the purpose of improvement of metrological characteristics the modernised variantof the helium magnetometer with automatic fine tuning of a phase in a feedback chain also has been developed.


Potassium Narrow Line Magnetometers combines the highest resolution (better than 1pT/Hz½!) with very high base line stability (better than 0.2 nT) and fast (~0.001 s) response. Being completely computerized, these devices are able to work with phase error suppressed down to 0.1nT in magnetic fields from 15,000 to 100,000nT with an accuracy of 0.1nT and tilt error as small as 0.03nT.


The HFS Magnetometer (the magnetometer using microwave transitions in Hyper-Fine Structure of the ground state of alkaline atoms) is expected to become the most perfect tool in aeromagnetic prospecting. It is the only instrument which is basically free from any restriction concerning its orientation in the magnetic field: it is inherently free from any tilt errors and "dead zones". These unique features are realized in a single-cell configuration while the only known by now approach to solving the problem bases on combination of several differently oriented units.


Potassium Vector Magnetometer combines the resolution of the quantum magnetometer with the possibility of continous measurement of transverse field components (inclination and declination) variations with 10pT accuracy. It was designed in co-operation with Jean Rasson (Institute Royal Meteorological, Centre de Physique du Globe, Dourbes, Belgium).


Cesium Vector Magnetometer-Variometer is more compact and robust device than Potassium one. Still, it allows to continously measure the variations of three orthogonal field components with resolution about 15pT and sample rate as high as 10 samples/sec.


The multi-sensor magnetometrical system based on a set of cesium self-oscillating sensors, is designed for experiments with ultracold neutrons in extra-low magnetic field:
1.Measuring an electric dipole moment of neutrons (EDM).
2.Search for neutron-mirror neutron oscillations.
3.Seach for macroscopic CP violating.


Several more types of scalar and vector magnetometers and gradientometers are developed or being developed, each of them intended to suit its own application field in a best way.





PUBLICATIONS

QUANTUM MAGNETOMETRY REVIEW
 
  1. Download article Download article E.B. Aleksandrov, A.K. Vershovskii. Modern radio-optical methods in quantum magnetometry. - Uspekhi Fizicheskikh Nauk 2009, v.179, n.6, pp.605-637.


ARTICLES
 
  1. P.P.Kuleshov, R.A.Zhitnikov. Self-generating on the larmor frequency quantum magnetometer. á.ó. ¹ 297321, âé, ¹13,1971.

  2. R.A.Zhitnikov, P.P.Kuleshov, A.I.Okunevich, N.A.Dovator, V.A.Azin. The self-generating magnetometer with an optical orientation of helium atoms 4îÅ. Geofiz.Apparat.V.50, p.3-8, 1972.

  3. N.A.Dovator, R.A.Zhitnikov, P.P.Kuleshov. The Helium self-oscillating magnetometer with phase autofine tuning. Geofiz.Apparat.¹56, s5-9, 1974.

  4. Download article E.B.Alexandrov, M.V.Balabas, A.S.Pazgalev, A.K.Vershovskii, N.N.Yakobson. Double-resonance atomic magnetometers: from gas discharge to laser pumping.-Laser Physics, v.6, no.2, p.244-251. (1996).

  5. Download article E.B.Alexandrov, M.V.Balabas, A.K.Vershovskii, A.S.Pazgalev. Multi-quantum radio-spectroscopy of atoms as applied to geomagnetic fields metrology (In Russian).-J.Tech.Phys, v.69, no.9, pp.27-30 (1999).

  6. Download article E.B.Alexandrov, M.V.Balabas, A.K.Vershovskii, and A.S.Pazgalev. A new model of quantum magnetometer: a single cell Cs-K tandem based on four-quantum resonance in 39K.- Tech. Phys. V.45 (7), pp.931-936 (2000).

  7. Download article A.K.Vershovski, A.S.Pazgalev, and E.B.Aleksandrov. The Design of a Lambda-HFS Magnetometer.-Tech. Phys. V.45, p.88 (2000).

  8. Download article E.B.Alexandrov, M.V.Balabas, V.N.Kulyasov, A.E.Ivanov, A.S.Pazgalev, J.L.Rasson, A.K.Vershovski and N.N.Yakobson. Three-component variometer based on a scalar potassium sensor.-Meas. Sci. Technol. Vol.15, pp.918-922 (2004).

  9. Download article Download article E.B.Alexandrov, M.V.Balabas, A.K.Vershovski, and A.S.Pazgalev. Experimental Demonstration of the Sensitivity of an Optically Pumped Quantum Magnetometer.-Tech. Phys., Vol. 49, No. 6, pp. 779-783 (2004).

  10. Download article Download article A.K.Vershovskii and E.B.Aleksandrov.-Phase Error Elimination in the Mx-Magnetometer and Controlling the Resonance Line Shape in an Unstable Field Using the Spin Precession Signal Invariant Mapping Technique.-Optics & Spectrosc., Vol.100, No.1, pp.12-14 (2006).

  11. Download article Download article A.K.Vershovskii.The new method of absolute measurement of three magnetic field components based on using of scalar optically pumped MX-magnetometer.-Optics & Spectrosc., Vol.101, No.2, pp.309-316 (2006)

  12. Download article Download article E. B. Aleksandrov, A. K. Vershovski, and A. S. Pazgalev, – Magnetometer based on a pair of symmetric transitions in the 87Rb hyperfine structure, – Technical Physics, v.51, no.7, pp. 919-923 (2006)

  13. Download article Download article A. K. Vershovski and A. S. Pazgalev, – Optically pumped quantum MX magnetometers: Digital measurement of the MX resonance frequency in a rapidly varying field, – Technical Physics, v.51, n.7, pp. 924–928 (2006)

  14. Download article A.K.Vershovskii, M.V.Balabas, A.E.Ivanov, V.N.Kulyasov, A.S.Pazgalev, and E.B.Aleksandrov. Fast 3-Component Variometer Based On A Cesium Sensor.- Publs. Inst. Geophys. Pol. Acad. 2007, C-99 (398)

  15. Download article A.Vershovskiy. Project Of Absolute Three-Component Vector Magnetometer Based On Quantum Scalar Sensor.- Publs. Inst. Geophys. Pol. Acad. 2007, C-99 (398).

  16. Download article Download article A. K. Vershovski and A. S. Pazgalev. - Optimization of Quality Factor of Optically Pumped Mx-resonance.- Technical Physics, 2008, v.78, No.5, pp. 116-124.